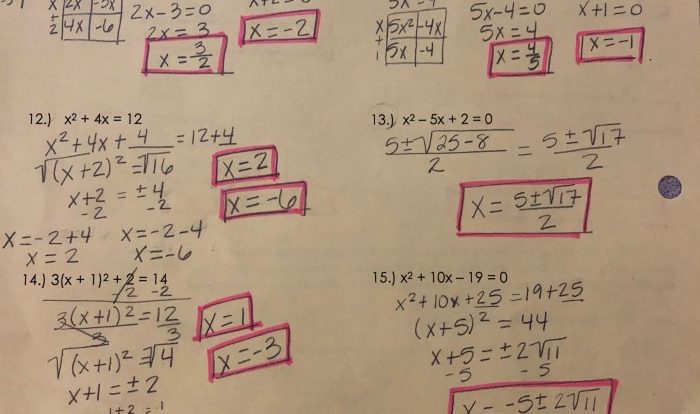Cheat sheet for geometry proofs – Step into the world of geometry proofs with our comprehensive cheat sheet, meticulously designed to empower you with the knowledge and techniques to conquer any proof with confidence.
Delve into the fundamentals, explore key theorems and postulates, and master various proof techniques. Practice makes perfect, so dive into our interactive exercises and solved examples to solidify your understanding.
Understanding Geometry Proofs
In geometry, a proof is a logical argument that demonstrates the truth of a mathematical statement. Proofs are essential for establishing the validity of geometric theorems and for developing a deep understanding of the subject.
Types of Geometry Proofs
There are several different types of geometry proofs, each with its own unique characteristics and applications.
- Two-column proofs: Two-column proofs are the most common type of geometry proof. They consist of two columns, one for the statements and one for the reasons. The statements are the mathematical assertions that are being proved, while the reasons are the logical arguments that support the statements.
- Paragraph proofs: Paragraph proofs are less formal than two-column proofs. They use complete sentences and paragraphs to explain the logical arguments that support the statements.
- Flowchart proofs: Flowchart proofs use a flowchart diagram to represent the logical arguments that support the statements. This type of proof is often used to prove complex theorems.
Importance of Understanding Geometry Proofs
Understanding geometry proofs is essential for several reasons.
- Proofs help to develop logical reasoning skills: Geometry proofs require students to use logical reasoning to support their arguments. This helps to develop their critical thinking skills and their ability to construct sound arguments.
- Proofs help to understand the structure of mathematics: Proofs help students to understand how mathematics is built upon a foundation of axioms and theorems. This helps them to appreciate the interconnectedness of mathematics and to see how different mathematical concepts are related.
- Proofs help to prepare students for higher-level mathematics: Proofs are an essential part of higher-level mathematics courses, such as calculus and linear algebra. Understanding proofs is essential for success in these courses.
Cheat Sheet for Geometry Proofs
Mastering geometry proofs requires a solid understanding of key concepts and theorems. This cheat sheet provides a comprehensive overview of essential information, organized for easy reference during proof-writing.
Key Concepts
- Definition:A statement that describes the essential characteristics of a mathematical object or concept.
- Theorem:A statement that has been proven true and can be used to prove other statements.
- Postulate:A statement that is assumed to be true without proof.
- Axiom:A statement that is considered self-evident and does not require proof.
- Converse:A statement that reverses the hypothesis and conclusion of a theorem.
- Inverse:A statement that negates the hypothesis and conclusion of a theorem.
- Contrapositive:A statement that negates the conclusion and hypothesis of a theorem.
Theorems and Postulates
Theorems and postulates are fundamental building blocks of geometry proofs. Theorems are statements that have been proven to be true, while postulates are statements that are assumed to be true without proof.
Both theorems and postulates are essential for constructing valid geometry proofs. Theorems provide the logical foundation for the proof, while postulates provide the basic assumptions that the proof relies on.
Fundamental Theorems
- Triangle Sum Theorem:The sum of the interior angles of a triangle is 180 degrees.
- Pythagorean Theorem:In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
- Angle Bisector Theorem:If a line bisects an angle of a triangle, then it divides the opposite side into two segments that are proportional to the lengths of the other two sides.
Fundamental Postulates
- Through any two points, there is exactly one line that can be drawn.
- A line segment can be extended indefinitely in either direction.
- All right angles are congruent.
| Theorem/Postulate | Statement |
|---|---|
| Triangle Sum Theorem | The sum of the interior angles of a triangle is 180 degrees. |
| Pythagorean Theorem | In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. |
| Angle Bisector Theorem | If a line bisects an angle of a triangle, then it divides the opposite side into two segments that are proportional to the lengths of the other two sides. |
| Through any two points, there is exactly one line that can be drawn. | A line segment can be extended indefinitely in either direction. |
| All right angles are congruent. | All right angles have the same measure. |
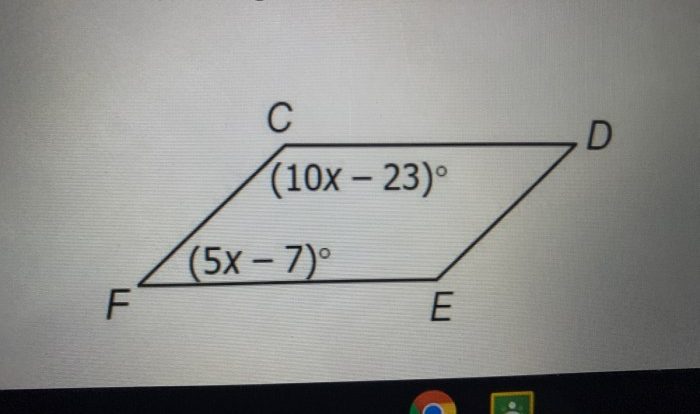
Examples
Theorems and postulates are used in a variety of geometry proofs. For example, the Triangle Sum Theorem can be used to prove that the sum of the interior angles of a quadrilateral is 360 degrees.
The Pythagorean Theorem can be used to prove that the diagonals of a rectangle are congruent.
Geometry proofs can be a pain, but thankfully there are cheat sheets to help you out. If you’re looking for a more lighthearted distraction, I recommend checking out meet the president zadie smith . Zadie Smith is a brilliant writer, and her essays are always insightful and entertaining.
Once you’re done with that, come back and conquer those geometry proofs!
The Angle Bisector Theorem can be used to prove that the perpendicular bisector of a line segment divides the line segment into two congruent segments.
Proof Techniques: Cheat Sheet For Geometry Proofs
In geometry, various proof techniques are employed to establish the validity of mathematical statements. These techniques provide a systematic approach to constructing logical arguments and deducing conclusions from given assumptions.
Direct Proof
A direct proof involves a step-by-step logical argument that leads directly to the desired conclusion. It proceeds from the given assumptions to the conclusion, without any additional assumptions or contradictions.
- Start with the given assumptions.
- Apply logical reasoning and geometric principles.
- Draw diagrams and label them accurately.
- State intermediate conclusions as needed.
- Continue the logical chain until the desired conclusion is reached.
Indirect Proof
An indirect proof, also known as a proof by contradiction, assumes the negation of the desired conclusion and leads to a contradiction. This forces the acceptance of the original conclusion.
- Assume the negation of the desired conclusion.
- Use logical reasoning and geometric principles.
- Show that the assumption leads to a contradiction.
- Conclude that the original assumption must be false.
- Therefore, the desired conclusion must be true.
Proof by Contrapositive
A proof by contrapositive involves proving the contrapositive of the original statement. The contrapositive is formed by swapping the hypothesis and conclusion of the original statement and negating both.
- State the original statement.
- Write the contrapositive of the original statement.
- Prove the contrapositive using a direct or indirect proof.
- Conclude that the original statement is true.
Proof by Cases
A proof by cases involves dividing the problem into several cases, each representing a different possibility. Each case is proven separately, and the final conclusion is drawn based on the results of all the cases.
- Identify all possible cases.
- Prove each case individually.
- Combine the results of all the cases.
- Draw the final conclusion.
Proof by Mathematical Induction
Proof by mathematical induction is used to prove statements about all natural numbers. It involves proving a base case for the smallest natural number, and then proving an inductive step that shows that if the statement is true for some natural number, then it must also be true for the next natural number.
- Prove the base case for the smallest natural number.
- Prove the inductive step: assume the statement is true for some natural number, and show that it must also be true for the next natural number.
- Conclude that the statement is true for all natural numbers.
Examples and Practice
To solidify your understanding of geometry proofs, let’s dive into some solved examples and interactive exercises. These will help you apply the techniques Artikeld in the cheat sheet and build your problem-solving skills.
Solved Examples, Cheat sheet for geometry proofs
We’ll provide step-by-step solutions to geometry proofs using different techniques, such as:
- Two-column proofs
- Indirect proofs
- Coordinate proofs
Interactive Exercises
Engage in interactive quizzes and exercises that test your ability to apply the cheat sheet’s principles. These exercises will:
- Challenge you with varying difficulty levels
- Provide instant feedback on your answers
- Help you identify areas for improvement
Organized Sections
For your convenience, the examples and exercises will be organized into sections based on difficulty level:
- Beginner
- Intermediate
- Advanced
Applications of Geometry Proofs
Geometry proofs are not just theoretical exercises but have practical applications in various real-world scenarios. They play a crucial role in architecture, engineering, and design, enabling professionals to make precise calculations and ensure the stability and functionality of structures.
Architecture
Architects use geometry proofs to determine the angles, lengths, and proportions of buildings. By applying theorems such as the Pythagorean theorem and the Law of Cosines, they can calculate the dimensions of structures, ensuring structural integrity and aesthetic appeal.
Engineering
Engineers rely on geometry proofs to design bridges, roads, and other infrastructure. They use proofs to determine the forces acting on structures, calculate stresses and strains, and ensure the safety and efficiency of their designs.
Design
Designers use geometry proofs to create visually pleasing and functional objects. By understanding the principles of geometry, they can create products that are both aesthetically appealing and ergonomically sound.
Quick FAQs
What is a geometry proof?
A geometry proof is a logical argument that demonstrates the validity of a geometric statement.
Why are proofs important in geometry?
Proofs provide a solid foundation for geometric knowledge, ensuring that theorems and postulates are not merely accepted as facts but are rigorously justified.
What are some common proof techniques?
Direct proof, indirect proof, proof by contradiction, and proof by cases are some widely used proof techniques in geometry.